RepresentationsOfGroupoidsInducedByMeasure

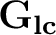
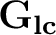
Definition 0.1 A groupoid representation induced by measure can be defined as measure induced operators or as operators induced by a measure preserving map in the context of Haar systems with measure associated with locally compact groupoids, $\mathbf{G_{lc}}$ . 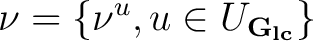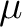Thus, let us consider a locally compact groupoid $\mathbf{G_{lc}}$ endowed with an associated Haar system $\nu = \left\{\nu^u, u \in U_{\mathbf{G_{lc}}} \right\}$ , and $\mu$ a quasi-invariant measure on $U_{\mathbf{G_{lc}}}$ . 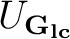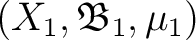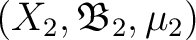Moreover, let $(X_1, \mathfrak{B}_1, \mu_1)$ and $(X_2, \mathfrak{B}_2, \mu_2)$ be measure spaces and denote by $L^0(X_1)$

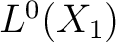
and

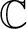
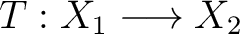
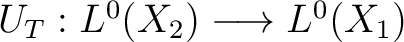
the corresponding spaces of measurable functions (with values in $\mathbb{C}$ ). Let us also recall that with a measure-preserving transformation $T: X_1 \longrightarrow X_2$ one can define an operator induced by a measure preserving map, $U_T:L^0(X_2) \longrightarrow L^0(X_1)$ as follows.

$\displaystyle (U_T f)(x):=f(Tx)\,, \qquad\qquad f \in L^0(X_2),\; x \in X_1$

Next, let us define 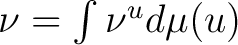$\nu = \int \nu^u d\mu (u)$ and also define 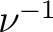$\nu^{-1}$ as the mapping 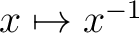$x \mapsto x^{-1}$ . With 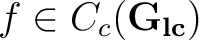$f \in C_c(\mathbf{G_{lc}})$ , one can now define the measure induced operator 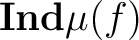$\textbf{Ind}\mu (f)$ as an operator being defined on 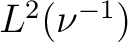$L^2(\nu^{-1})$ by the formula:

$\displaystyle \textbf{Ind}\mu (f)\xi(x)= \int f(y) \xi(y^{-1}x)d\nu^{r(x)}(y) = f * \xi(x)$

and also that $\textbf{Ind}\mu$ is a proper representation of $C_c(\mathbf{G_{lc}})$ , in the sense that the latter is usually defined for groupoids.